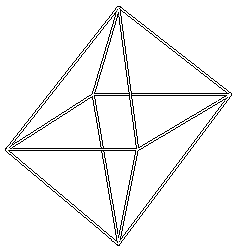
When we perceive the Awesome, its capacity of expression will often "carry us away", allowing us to become exalted by the Beautiful, the Sublime, or even the merely attractive. This exaltation, this metaphorical "carrying away" into what is being expressed, is called Transport. When I, personally, see what I hold to be a beautiful mathematical object—say, one of the Platonic Solids—I see it as expressing at least two ideas or realms. First, it expresses the realm of Mathematics itself, in this specific case Geometry. The regularity of the Platonic Solid, the fact that all its edges are the same length and all its faces the same size and shape entails the identity of the unit of measurement, both linear and angular. But of course, the ideas of 'unit', 'identity', and 'measurement' of line and angle are first and foremost mathematical ideas. They exist primarily with respect to the field of Geometry. The Platonic Solid, thus, expresses for me the whole realm of Geometry, and by association (at least) the whole of Mathematics. Second, because of my knowledge of the relationship between Geometry and SpaceTime—or more precisely, because I know that SpaceTime is a kind of Geometry—the Platonic Solid says something to me about the actual SpaceTime that I live in. If our SpaceTime had a different Geometry, there would be a different set of "Platonic Solids", since—as Euler and others have shown—the number and form of the Platonic Solids—or Regular Polyhedra, as they are called by mathematicians—is determined by the geometrical properties of SpaceTime. Different properties of SpaceTime determine different possibilities of physical shape.