Fibonnaci’s
Sequence and the Golden Proportion
FFFFFFFFFFFFFFFFFFFFFFFFFFFF

FFFFFFFFFFFFFFFFFFFFFFFFFFFF
One
part in particular sticks in my mind from that old animated movie Donald in MathMagic
Land and it is a constant irritation to me that my subtle allusions to the
movie and the “Golden Proportions” meet with blank stares from my friends and
family; subtlety is the basis for humor and I am a subtle and witty guy. Anyway, what amazed me about Donald’s
discussion with Pythagoras regarding the Golden Proportion is the Proportion’s
relevance in the world. Pythagoras
danced along violin strings explaining the relationship between the tonal
scales and the Proportion …Vivid yellow rectangles were superimposed snugly
over the Proportion and other Greek structures of the day, which then dissolved
under the rectangles to be replaced by the paintings of Leonardo da Vinci, Seurat, and Mondrian. I was amazed. With the Golden Rectangle placed directly on
top of these architectural icons and well-known paintings, the repetitiveness
and prevalence of these proportions was clear.
Was this done on purpose? Were
the proportions chosen first and then the building built and the paintings
painted? Or was this simply an inherent
characteristic of “beauty” that artists unknowingly produced again and again?
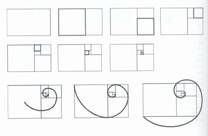
The Golden Rectangle has been described as one of the
most visually satisfying geometric shapes ever discovered. It can be derived repeatedly without
mathematics by simply using the relationships of the sides. However, there is a clear mathematical
relationship and proportion here that simply explodes in its limitless
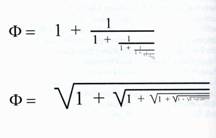
possibilities as more and more is discovered about it. When the length of the rectangle is divided
by the width, the answer is 1.618. If
the width is divided by the length, the answer is 0,618. Big deal, huh? Not so, Grasshopper. Solve for x where
1/x=x+1. x=0.618. The Golden Rectangle can be increasingly
enlarged, as Donald seemed to realize, and then superimposed exactly over the
logarithmic spirals of a Nautilus or the petals of a flower.
The Golden Rectangle and Proportion is linked
inextricably with the Fibonacci Series, which is the complementary view of the
Golden Proportion. It is called the
Fibonacci Series after Leonardo of Pisa, alias Leonardo Fibonacci, born in
1175, whose great book The Liber Abaci (1202), on arithmetic, was a standard work
for 200 years and is still considered the best book written on arithmetic. Fibonacci discovered the series of numbers
beginning: 0, 1, 1, 2, 3, 5, 8, 13, 21,
34, 55, 89, 144, etc.; add the last two numbers to get the next. Notice that the ratios of successive
Fibonacci numbers Fn/Fn-1, approaches the Golden
Proportion as n approaches infinity!
The Fibonacci numbers form the best whole number approximations to the
Golden Number.
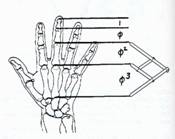
These numbers are replete throughout nature. Dentists know that as you proceed from the
larger teeth at the front of the mouth to the smaller in back, you find in them
the relationships of the Fibonacci numbers:
the Gold Proportions. Plastic
surgeons use the Golden Proportion when attempting to achieve the ever-so
subtle and seemingly indefinable quality of physical beauty in a human
being. Golden Proportion calipers, which
can be autoclaved for sterility, are available to these surgeons in order to
more quickly find this relationship in the operating room. If you count the opposing spirals found on
the base of a pinecone, you will always find them to be 5 or 8, or 8 and 13,
successive Fibonacci numbers; so too with a pineapple, or a daisy. The technical term for this study of the
arrangements of branches, leaves and of seed heads, in plants is phyllotaxis. The
arrangement of leaves is the same for seeds and petals. All are placed at 0.618034… leaves, (seeds
and petals) per turn. In terms of degrees,
this is 0.618034 of 360o.
This is more than just fascinating happenstance. If there are 1.618… leaves per turn (or,
equivalently, 0.618…turns per leaf), then we have the best arrangement whereby
each leaf gets the maximum exposure to light, casting the least shadow on the
others. This also gives the best
possible area exposed to falling rain so that the rain is directed back along
the leaf and down the stem to the roots.
For flowers or petals, it gives the best possible exposure to insects to
attract them for pollination. The whole
of the plant seems to produce its branches, leaves, flowerhead
petals and then seeds based upon the Golden Number. The discoveries of the occurrences of this
Proportion and this Series continue today.
There is a large Society devoted to Fibonacci in
(from
ExamKrackers, Inc.
copyright 2002, p. 84)
(images
from The Beginner’s Guide to Constructing
the Universe)